Schematic Diagram Of Turbojet Engine

VIII. Ideal Cycle Analysis of Aircraft Gas Turbine Engines

A.   Introduction

In thermodynamics we represented a gas turbine engine using a Brayton cycle, as shown in Figure 8.1, and derived expressions for efficiency and work as functions of the temperature at various points in the cycle.� In this section we will perform �ideal cycle analysis�, which is a method for expressing the thrust and thermal efficiency of engines in terms of useful design variables.� If you take a further course in propulsion, this ideal cycle analysis will be extended to take account of various inefficiencies in the different components of the engine��that type of analysis is called non-ideal cycle analysis.

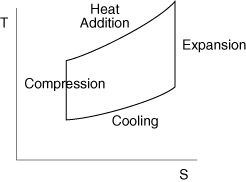
Figure 8.1 Schematic of a Brayton cycle.

1. Objective of ideal cycle analysis

Our objective is to express thrust, T, and thermal efficiency, h (or alternatively I) as functions of 1) typical design limiters, 2) flight conditions, and 3) design choices so that we can analyze the performance of various engines.� The expressions will allow us to define a particular mission and then determine the optimum component characteristics (e.g. compressor, combustor, turbine) for an engine for a given mission.� Note that ideal cycle analysis addresses only the thermodynamics of the airflow within the engine.� It does not describe the details of the components (the blading, the rotational speed, etc.), but only the results the various components produce (e.g. pressure ratios, temperature ratios).� In Section IX we will look in greater detail at how some of the components (the turbine and the compressor) produce these effects.

Q21 (PDF)
Q22 (PDF)

2. Notation and station numbering

����

Figure 8.2 Gas turbine engine station numbering.

Notation:

�����������

����������� ������

����������� ������� ()

Stagnation properties, T_T & P_T, are more easily measured quantities than static properties (T and p).� Thus, it is standard convention to express the performance of various components in terms of stagnation pressure and temperature ratios:

p � total or stagnation pressure ratio across component (d, c, b, t, a, n)

t � total or stagnation temperature ratio across component (d, c, b, t, a, n)

where d= diffuser (or inlet), c= compressor, b= burner (or combustor), t= turbine, a= afterburner, and n=nozzle.

3. Ideal Assumptions

����� 1)�� Inlet/Diffuser:� p_d = 1, t_d = 1 (adiabatic, isentropic)

����� 2)�� Compressor or fan:� t_c = p_c ^g-1/g ,� t_f = p_f ^g-1/g

����� 3)�� Combustor/burner or afterburner:� p_b = 1, p_a = 1

����� 4)�� Turbine:� t_t = p_t ^g-1/g

����� 5)�� Nozzle:� p_n = 1, p_n = 1

Check out the Thermo notes for a refresher on cycle analysis done in the fall - GO!

B.   Ideal Cycle Analysis Example: Turbojet Engine

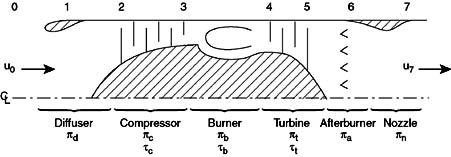
Figure 8.3 Schematic with appropriate component notations added.

Methodology:

1)      Find thrust by finding u_exit/u_o in terms of q_o, temperature ratios, etc.

2)      Use a power balance to relate turbine parameters to compressor parameters

3)      Use an energy balance across the combustor to relate the combustor temperature rise to the fuel flow rate and fuel energy content.

First write-out the expressions for thrust and I:

������������� where f is the fuel/air mass flow ratio

�������� (can neglect fuel)

That is the easy part!� Now we have to do a little algebra to manipulate these expressions into more useful forms.

First we write an expression for the exit velocity:

Noting that

We can write

Thus

�� (*)

Which expresses the exit temperature as a function of the inlet temperature, the Mach number, and the temperature changes across each component.� Since we will use this expression again later we will mark it with an asterisk (*).

We now write the pressure at the exit in a similar manner:

and then equate this to our expression for the temperature (*)

�� (**)

and again label it (**) for use later as we do the following expression:

�� (***)

We now continue on the path to our expression for u₇/u_o.

Therefore

Now we have two steps left.� First we write t_c in terms of t_t, by noting that they are related by the condition that the power used by the compressor is equal to the power extracted by the turbine.� Second, we put the burner temperature ratio in terms of the exit temperature of the burner, (T_T4 or more specifically q_t=T_T4/T_o) since this is the hottest point in the engine and is a frequent benchmark used for judging various designs.

The steady flow energy equation tells us that

�����������

Assuming that the compressor and turbine are adiabatic, then

����� = - rate of shaft work done by the system = rate of shaft work done on the system

Since the turbine shaft is connected to the compressor shaft

���� assuming and C_p are the same

This can be rewritten as

����������� �������� where��������������

so�

������������ or��������

That was the first step � relating the temperature rise across the turbine to that across the compressor.� The remaining step is to write the temperature rise across the combustor in terms of q_t=T_T4/T_o.

����

and for an engine with an afterburner

Now substituting our expressions for t_b, and t_t into our expression for u₇/u_o, and finally into the first expression we wrote for thrust, we get:

������� Specific thrust for a turbojet

which is what we were seeking, an expression for thrust in terms of important design parameters and flight parameters:

With algebra�

We may write this write in another form which is often used

�����������

Our final step involves writing the specific impulse and other measures of efficiency in terms of these same parameters.� We begin by writing the First Law across the combustor to relate the fuel flow rate and heating value of the fuel to the total enthalpy rise.

and������

���������������� where again, f is the fuel/air mass flow ratio

So the specific impulse becomes

Specific Impulse for an ideal turbojet

where I is expressed in terms of typical design parameters, flight conditions, and physical constants

Similarly, we can write our overall efficiency, h_overall

�����������

����������� ����������������� or��������

The ideal thermal efficiency is

�����������

and the propulsive efficiency can be found from h_prop = h_overall / h_thermal

We can now use these equations to better understand the performance of a simple turbojet engine.� We will use the following parameters (with g=1.4):

Mach number	Altitude	Ambient Temp.	Speed of sound
0	Sea level	288K	340m/s
0.85	12km	217K	295m/s
2.0	18km	217K	295m/s

Stanford has a great standard atmosphere calculator that can be used to find conditions at any altitude - GO!

Note it is more typical to work with the compressor pressure ratio (p_c) rather than the temperature ratio (t_c) so we will substitute the isentropic relationship:

����������t_c = p_c ^g-1/g

into the equations before plotting the results.

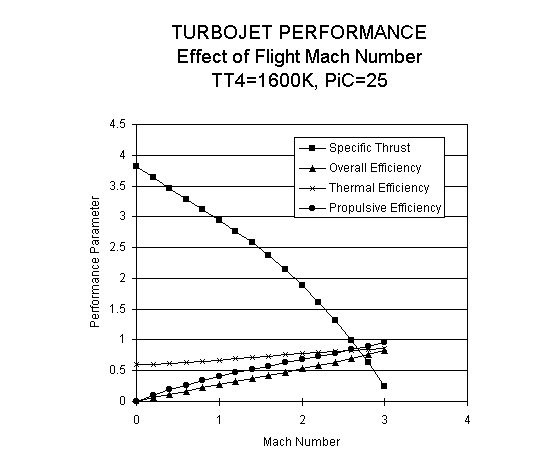
Figure 8.4 Performance of an ideal turbojet engine as a function of flight Mach number.

�

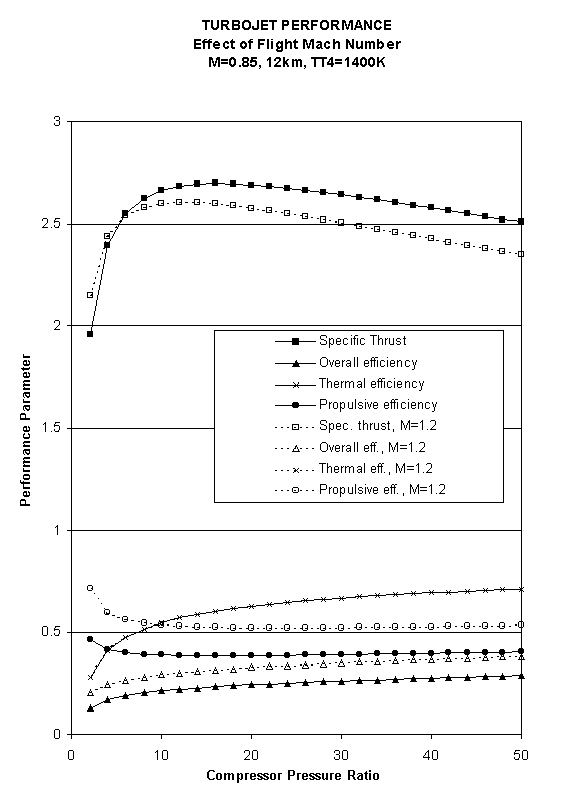
Figure 8.5 Performance of an ideal turbojet engine as a function of compressor pressure ratio and flight Mach number.

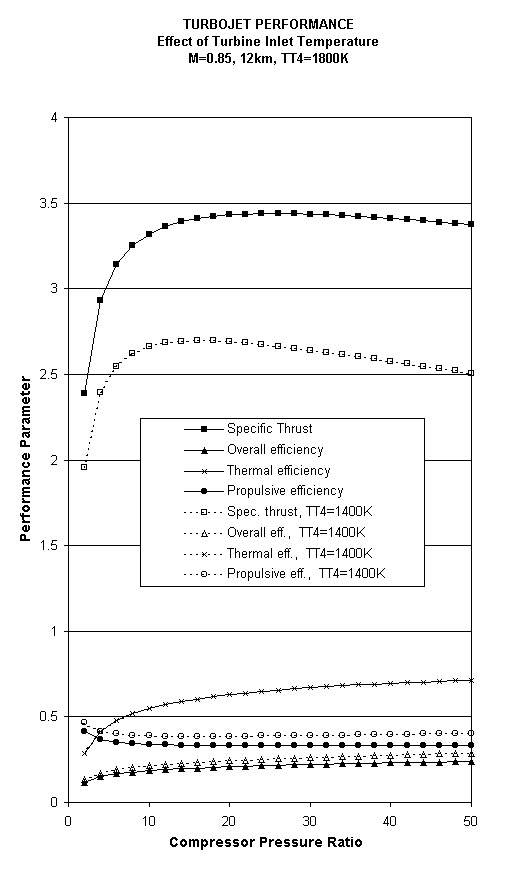
Figure 8.6 Performance of an ideal turbojet engine as a function of compressor pressure ratio and turbine inlet temperature.

Homework P7 (PDF)

Posted by: teganteganschaetzlee0267903.blogspot.com

Source: https://ocw.mit.edu/ans7870/16/16.unified/propulsionS04/UnifiedPropulsion8/UnifiedPropulsion8.htm

Share :